Have you ever looked at a triangle and wondered if it was special? Did you know that within the seemingly simple geometry of a right triangle lies a powerful mathematical relationship that unlocks a world of possibilities? The Pythagorean Theorem, a timeless principle that connects the sides of right triangles, has been shaping our understanding of geometry and its applications for millennia. This theorem, along with its converse, is not just a chapter in your math textbook, but a fundamental concept that underpins our understanding of the world around us – from building sturdy structures to navigating vast oceans.
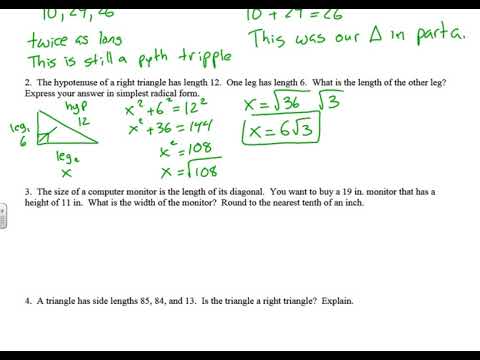
Image: www.youtube.com
This journey will take us through the heart of the Pythagorean theorem, its converse, and their profound impact on our world. We’ll explore the history of its discovery, delve into the mathematical reasoning behind it, and discover how it is applied in everyday situations. Get ready to be amazed by the power of a simple equation that has impacted human ingenuity for centuries.
A Glimpse into History: The Legacy of a Theorem
The Birth of a Powerful Concept
The Pythagorean Theorem, named after the ancient Greek mathematician Pythagoras, is a fundamental concept in geometry. It states that in a right triangle, the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. This relationship, expressed by the equation a2 + b2 = c2, where ‘a’ and ‘b’ are the lengths of the shorter sides (legs) and ‘c’ is the length of the hypotenuse, seems simple enough. But its discovery revolutionized how we perceive and understand triangles.
While often attributed solely to Pythagoras, historical evidence suggests that the theorem may have been known and utilized in various cultures prior to his time. Ancient civilizations like the Babylonians and Egyptians had developed methods for calculating the sides of right triangles. However, Pythagoras is believed to have developed a more formal proof of the theorem, contributing significantly to the advancement of mathematical understanding.
Unraveling the Mystery: Understanding the Theorem

Image: lessonlibraryyadiel77.z19.web.core.windows.net
Visualizing the Connection
To grasp the essence of the Pythagorean theorem, let’s visualize it. Imagine a right triangle with legs of length 3 and 4 units. Calculating the square of each side, we get 32 = 9 and 42 = 16. The sum of these squares, 9 + 16 = 25, represents the square of the hypotenuse. Finding the square root of 25, we get 5, the length of the hypotenuse.
This simple example demonstrates the relationship between the sides of a right triangle and how the Pythagorean theorem allows us to calculate the unknown side if we know the lengths of the other two sides.
Proofs of the Theorem: A Tapestry of Mathematical Elegance
The Pythagorean theorem has been proven in various ways throughout history, reflecting the ingenuity of mathematicians across different eras. One of the most common proofs involves dividing a square into four congruent right triangles and a smaller square. By rearranging these shapes and applying basic geometric concepts, we can derive the relationship a2 + b2 = c2.
Other proofs rely on different geometric approaches, highlighting the diverse perspectives through which this fundamental concept can be analyzed. The beauty of these proofs lies in their elegance and mathematical rigor, showcasing the power of deductive reasoning in uncovering deeper truths.
Beyond the Triangle: The Converse of the Pythagorean Theorem
Flipping the Logic: A New Perspective
Just as there’s a converse to a conditional statement in logic, there’s a converse to the Pythagorean theorem in geometry. The converse states that if the square of the longest side of a triangle is equal to the sum of the squares of the other two sides, then the triangle is a right triangle. In essence, the converse uses the theorem’s relationship to determine the type of triangle, rather than calculating a side.
Applications of the Converse: Building on the Foundation
The converse of the Pythagorean theorem is crucial in many applications, especially in fields like construction, surveying, and navigation. For example, when building a rectangular frame, we can use the converse to ensure that the corners are right angles. By measuring the sides of the frame and verifying that the Pythagorean relationship holds true, we can guarantee a perfect rectangular shape. This ensures stability and maximizes efficiency in construction projects.
Similarly, surveyors use the converse to determine if a piece of land is rectangular or if a structure is properly aligned. By measuring the distances between specific points on the land or the structure, they can apply the converse of the Pythagorean Theorem to ascertain if the angles are right angles.
The Power of the Pythagorean Theorem: A World of Applications
From Bridges to Satellites: Real-World Triumphs
The Pythagorean Theorem extends beyond theoretical geometry and plays a vital role in shaping the world around us. Here are some compelling examples of its real-world applications:
- Construction and Engineering: The theorem is fundamental in designing and building structures, from simple buildings to towering bridges. By calculating distances and angles, engineers ensure structural integrity and safety, optimizing materials and efficiency.
- Navigation: Whether you’re navigating a ship across oceans or a plane through the skies, the Pythagorean theorem is essential. It helps determine distances, angles, and trajectories, enabling precise navigation and safe travel.
- Computer Graphics: In the world of computer graphics and animation, the Pythagorean theorem is used to calculate distances and positions of objects, enabling realistic simulations and captivating visual effects.
- Astronomy: The theorem is used in astronomical calculations to determine the distances between celestial bodies. It helps us understand the vastness of the universe and the intricate relationships between stars, planets, and galaxies.
Unveiling the Hidden: The Theorem and the Human Mind
The Pythagorean Theorem has not only shaped our understanding of the physical world but has also played a key role in shaping human intellect. It serves as a testament to the power of logical reasoning and deductive thinking, inspiring mathematicians, scientists, and inventors. The theorem’s elegance and universality have stimulated centuries of exploration, pushing the boundaries of human knowledge.
Homework 1 Pythagorean Theorem And Its Converse
Conclusion: A Timeless Legacy
The Pythagorean Theorem, with its converse, is far from just a formula from your math textbook. It is a powerful tool that has shaped civilization and continues to drive progress in various fields. From the foundations of our buildings to the vastness of space, the theorem demonstrates the interconnectedness of mathematics, geometry, and the world around us. As you delve further into this fascinating realm, remember the enduring power of the Pythagorean Theorem and its ability to illuminate the hidden wonders of our universe.
Explore further resources, discover its applications in different fields, and share your experiences with the Pythagorean theorem. This journey through the world of right triangles has just begun!






